Rooks Problem -- from Wolfram MathWorld

The rook is a chess piece that may move any number of spaces either horizontally or vertically per move. The maximum number of nonattacking rooks that may be placed on an n×n chessboard is n. This arrangement is achieved by placing the rooks along the diagonal (Madachy 1979). The total number of ways of placing n nonattacking rooks on an n×n board is n! (Madachy 1979, p. 47). In general, the polynomial R_(mn)(x)=sum_(k)r_k^((m,n))x^k whose coefficients r_k^((m,n)) give the

In how many ways can you arrange eight queens on a standard chessboard in such a way that none of them is attacking any other? - Quora

Wolfram MathWorld - Murat Alper

Aggregation and Tiling as Multicomputational Processes—Stephen Wolfram Writings

Schaum's Outline of Mathematica, 2ed (Schaum's Outlines) 2, Don, Eugene

Rook Polynomials: A Straight-Forward Problem – Feature Column

What is the minimum number of Queens with which you can cover every square of chess board? Also show their arrangement. - Quora

In how many ways can you arrange eight queens on a standard chessboard in such a way that none of them is attacking any other? - Quora

discrete mathematics - n-rooks n-colors problem - Mathematics Stack Exchange

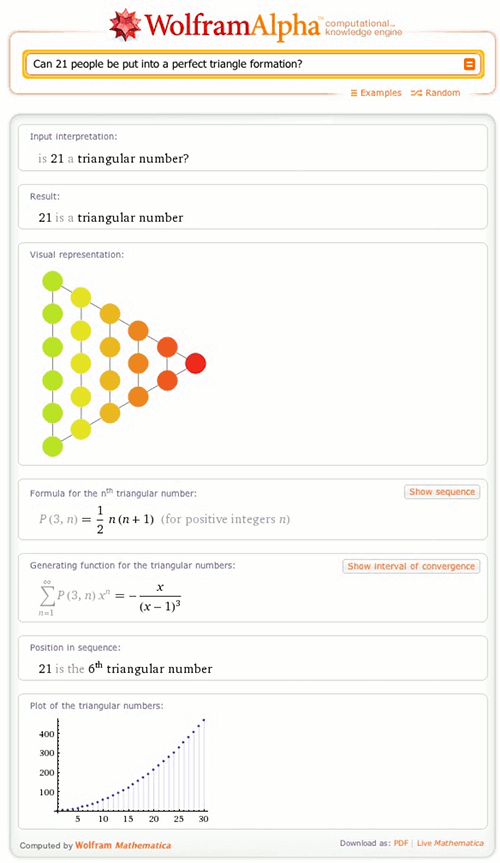
Posts Tagged with 'Mathematics'—Wolfram